RetrieverBreeder
- Project name: RetrieverBreeder
- Class: 4IT496 (WS 2015/2016)
- Author: Bc. Lucie Pokorná
- Model type: Discrete-event simulation
- Software used: SimProcess, trial version
Recommended work outline:
Problem definition - a description of the situation you solve (i.e. the task) Method - the discussion of possible solutions, the selection of method and tools for the solution, reasons for such choice (why the selected methods and tools are the best for the problem) Detailed description of the method, including parameters, ranges, schemes, model limitations, etc. The description must be detailed enough that anybody could replicate the experiment event without your model source codes. Results - list of results, their analysis, interpretation and evaluation. Conclusion - how the problem was solved Citations Model source code (xls, spm, nlogo, mdl, etc. file)
Contents
[hide]Problem definition
Simulation should answer the question how many female dogs is optimal to keep for satisfying the demand for golden retriever puppies.
Detailed problem definition
he goal of the simulation is to simulate the whole simplified process to find the optimal amount of female golden retriever dogs owned and/or kept regarding all given variables and facts. Goal is to only have the ideal number of breeding dogs capable to fulfill the given birth giving plan and indirectly let the owner of a cattery satisfy the demand.
Method
When first seeing the SIMPROCESS possibilities and observing the way to show the simulation running, an idea of pet breeding simulation almost immediately came to mind. Such a simulation compound of the generating (literally generating in this case) an entity - puppy delivery, delay - puppy growth and then disposing the entity - either finding a match with a corresponding demand (a waiting customer), offering and older puppy for lower price, or just keeping the particular one in a cattery - is exactly the discrete-event type of simulation that could be shown quite transparently, comprehensible yet clearly enough using this simulation tool.
While the simulation has been conducted, no significant restrictions were found using just a trial version of the program. Few not that necessary activities had to be cut and the rest of the simulation optimized to make sure that the limit for a number of activities is not depriving the simulation of possibly interesting results.
Model
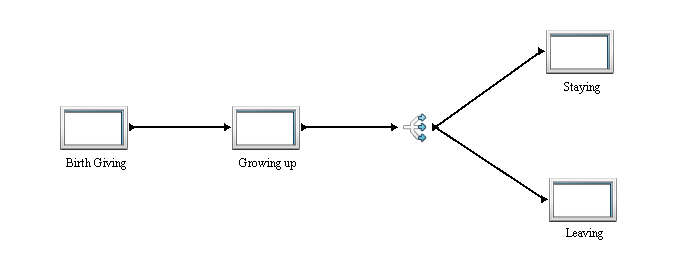
The simulation consists of 4 processes:
- "Birth giving" - puppy generating
- "Growing Up" - delay activity
- "Staying" - the cattery owner decides to keep a puppy
- "Leaving" - ideally a customer picks up a puppy, non ideally puppy is left "unwanted" for a longer period of time and has to be later sold for a lowered price or given entirely
Just one of the processes - the "Leaving" process - contains most of the activities used starting from the probability based division of puppy gender, customer decision making situation and handling an occasional exception - the case when there is no demand for a particular puppy and not even the cattery owner desires to keep it.
The model is based on real data gathered in the stated sources.
The simulation is set to be run in numerous iterations (replications). The more breeding female dogs the cattery owns, the more birth giving occasions there are. For the purposes of this simulation there is no need to simulate breeding dogs in any way, the only thing that is important is the recurrences of such events such is birth givings. It has been decided that each replication will simulate just a one litter had by one female breeding dog. In average, female golden retriever female is capable to give birth once a year and a half when the dog's health and well being is considered a number one priority. Simulation is set to show just a one such cycle. The lasting of a whole simulation including several replications is set to be an exact year and a half. That means that the number of replications equals to number of female breeding dogs within 18 months and actual breeding dogs as en entity or a resource may now be omitted in the simulation.
The distribution function for the demand is set to be invariable in a several runs of a simulation set period of time for a slight simplification. From a personal experience, demand for pedigreed puppies from a particular cattery changes quite a lot, yet the average remains at a very similar level, no matter how many puppies were sold in the past. It is possible and recommendable to adjust the value higher (lower) in a consequent time period - when the time simulated in a simulation passes - when the average demand grows (decreases) based on the actual demand counts have been observed and noted.
Entities
Resources
Processes
Results