Difference between revisions of "Pension System Czech Republic"
(→Variables) |
(→Variables) |
||
| Line 43: | Line 43: | ||
*Childrens: Represents the population aged 0-19 and serves as new entrants to the future workforce. | *Childrens: Represents the population aged 0-19 and serves as new entrants to the future workforce. | ||
**birth: Represents the number of children entering the stock of "Children" annually, driven by the fertility rate. | **birth: Represents the number of children entering the stock of "Children" annually, driven by the fertility rate. | ||
| − | **grown up: Represents the transition of individuals from "Children" to "Working-age Population," determined by the growing up rate (later | + | **grown up: Represents the transition of individuals from "Children" to "Working-age Population," determined by the growing up rate (later the figure reflects the number of births before 20 years). |
*Working-age Population: Population aged 20-64, representing workers contributing to the pension system. | *Working-age Population: Population aged 20-64, representing workers contributing to the pension system. | ||
**growing up: Transition from "Children" stock into "Working-age Population." | **growing up: Transition from "Children" stock into "Working-age Population." | ||
| Line 59: | Line 59: | ||
'''Economic variables''' | '''Economic variables''' | ||
*Average Gross Wage: Impacts the total contributions collected for the pension system. | *Average Gross Wage: Impacts the total contributions collected for the pension system. | ||
| + | **gross wage increase | ||
| + | **gross wage reduction | ||
| + | *Average Pension | ||
| + | ** valorization of pension: | ||
| + | ** pension reduction | ||
'''Fiscal variables''' | '''Fiscal variables''' | ||
| Line 65: | Line 70: | ||
**pension | **pension | ||
*yearly contribution: Determined by the number of workers, average gross wage, contribution rate and unemployment rate. | *yearly contribution: Determined by the number of workers, average gross wage, contribution rate and unemployment rate. | ||
| + | *enemployment rate | ||
'''Random variables''' | '''Random variables''' | ||
| Line 74: | Line 80: | ||
'''Auxiliary variables''' | '''Auxiliary variables''' | ||
| − | fertility rate history: | + | *fertility rate history: Historical record of fertility rates to ensure accurate projections of future adult populations after 20 years. |
| − | population history: Tracks past population levels for precise calculations in future aging. | + | *population history: Tracks past population levels for precise calculations in future aging (after 20 years). |
| − | simulation time: Tracks the current simulation cycle for transitioning between estimated and precise aging. | + | *simulation time: Tracks the current simulation cycle for transitioning between estimated and precise aging. |
| + | *population: Represents the total population at any simulation step, serving as the sum of all age cohorts. | ||
Revision as of 15:55, 8 January 2025
Contents
[hide]Introduction
Simulation of pension reform in the Czech Republic: Analysis of long-term sustainability
What will be simulated:
A system dynamics model of the Czech pension system that simulates the long-term financial sustainability under varying demographic, economic, and policy scenarios. The simulation will project future balances of the pension system, incorporating the flows of revenues (social insurance contributions) and expenditures (pension payouts)
Problem definition
The main goal is to analyze how different pension reform strategies (e.g., adjusting retirement age, altering contribution rates, changing the indexation formula of pensions) will affect the long-term stability of the Czech pension system. The simulation aims to identify specific policy levers and thresholds that ensure the pension system’s financial equilibrium over a multi-decade horizon, despite changing demographic and economic conditions.
Method
The System Dynamics method was chosen for its ability to model complex systems with multiple influencing variables, inflows, and outflows. Alternative approaches, such as Monte Carlo analysis or agent-based modeling in NetLogo, were deemed unsuitable for this case as they cannot adequately capture the dynamic interactions and feedback loops between demographic, economic, and policy variables. Vensim PLE was selected as the simulation environment due to its accessibility and support for this type of modeling.
Model
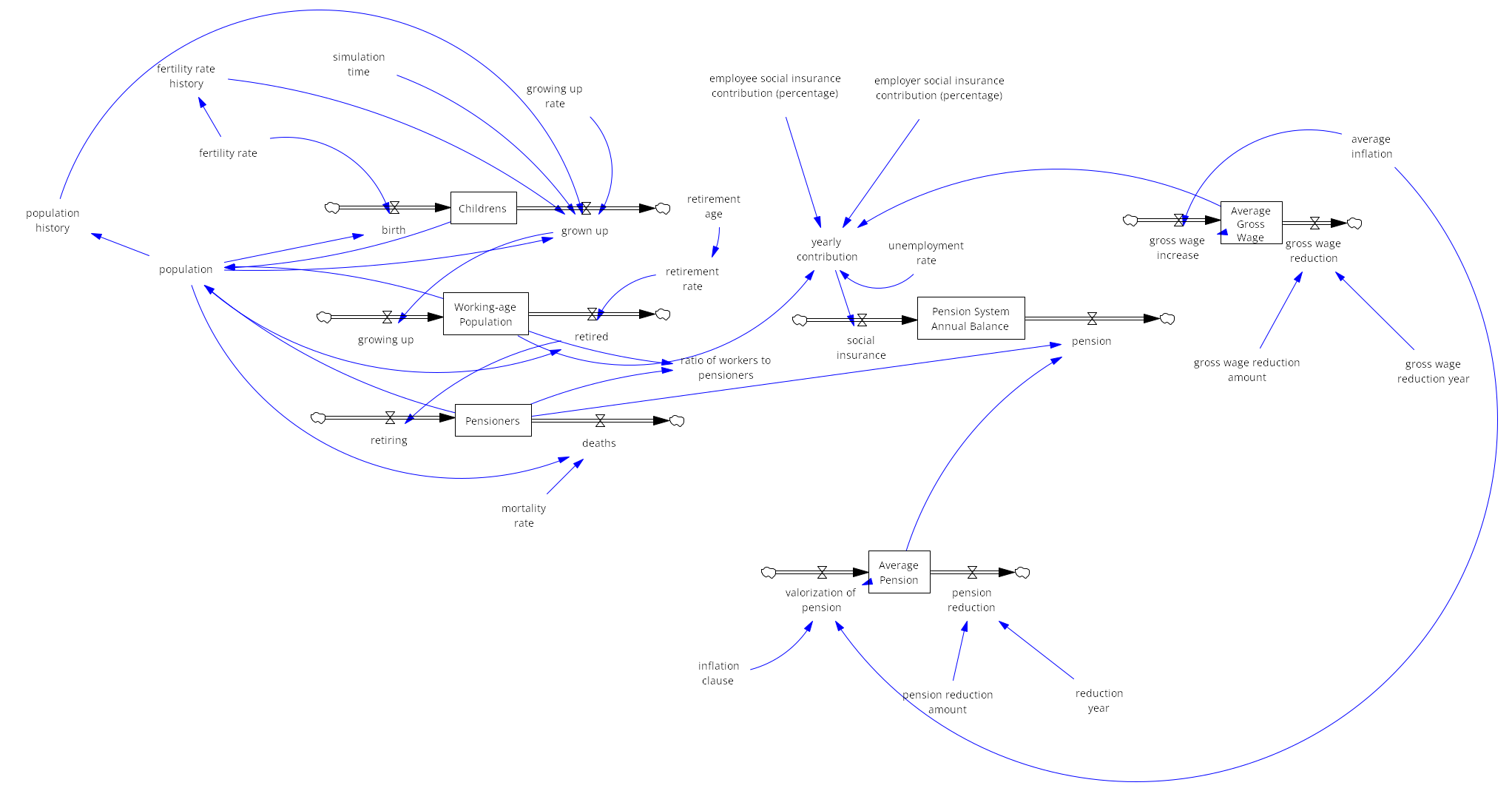
The model represents the dynamics of the Czech pension system, divided into key components: population structure, contributions to the pension system, and pension expenditures.
Population structure
The population is categorized into children (ages 0–19), working-age population (ages 20–64), and pensioners (65+ years). Children grow up and transition into the working-age population, which contributes to the pension system through employment. Over time, individuals retire and become pensioners, withdrawing from the pension system. The retirement age determines the rate at which the working-age population transitions to retirement, with a higher retirement age reducing the retirement rate and vice versa.
Contribution to the pension system
The working-age population contributes to the system through social insurance contributions, based on the average gross wage. Contributions come from both employees and employers, while unemployment reduces the total contributions collected. The model assumes that the gross wage grows annually, at least partially indexed to inflation (e.g., one-third of the inflation rate).
Pension expenditures
Pensioners withdraw funds from the system based on the average pension, which is periodically adjusted through pension valorization. The inflation clause determines the extent to which pensions are indexed to inflation, ensuring their real value remains stable over time.
External adjustments
The model includes variables for one time reductions in both the average gross wage and the average pension in a specific year. These adjustments simulate policy interventions (e.g., government mandated pension reductions) or external shocks (e.g., an economic crisis) that impact wages or pensions.
This dynamic interaction between population groups, contribution flows, and pension expenditures allows for the simulation of various policy scenarios, illustrating the long-term sustainability of the pension system.
Variables
Population variables
- Childrens: Represents the population aged 0-19 and serves as new entrants to the future workforce.
- birth: Represents the number of children entering the stock of "Children" annually, driven by the fertility rate.
- grown up: Represents the transition of individuals from "Children" to "Working-age Population," determined by the growing up rate (later the figure reflects the number of births before 20 years).
- Working-age Population: Population aged 20-64, representing workers contributing to the pension system.
- growing up: Transition from "Children" stock into "Working-age Population."
- retired: Represents the number of individuals leaving the working-age population to become pensioners, driven by the retirement rate.
- Pensioners: Population aged 65 and above, representing beneficiaries of the pension system.
- retiring: Transition of individuals from "Working-age Population" to "Pensioners."
- deaths: Represents the number of pensioners leaving the stock due to mortality, driven by the mortality rate.
Policy variables
- retirement age: Defines the age at which individuals become eligible for a pension (higher retirement age lower retirement rate and vice versa).
- employee social insurance contribution (percentage)
- employer social insurance contribution (percentage)
- inflation clause
Economic variables
- Average Gross Wage: Impacts the total contributions collected for the pension system.
- gross wage increase
- gross wage reduction
- Average Pension
- valorization of pension:
- pension reduction
Fiscal variables
- Pension System Annual Balance
- social insurance
- pension
- yearly contribution: Determined by the number of workers, average gross wage, contribution rate and unemployment rate.
- enemployment rate
Random variables
- fertility rate: Simulates uncertainty in future workforce size, based on historical data.
- mortality rate: Models variations in life expectancy and death rates.
- average inflation: Captures economic variability using stochastic projections.
- retirement rate: Reflects the variation in the number of people retiring annually.
- growing up rate: Simulates the transition of children (aged 0-19) into the working-age population, based on fertility patterns for the first 20 years and precise simulation data thereafter.
Auxiliary variables
- fertility rate history: Historical record of fertility rates to ensure accurate projections of future adult populations after 20 years.
- population history: Tracks past population levels for precise calculations in future aging (after 20 years).
- simulation time: Tracks the current simulation cycle for transitioning between estimated and precise aging.
- population: Represents the total population at any simulation step, serving as the sum of all age cohorts.
Economic adjustment variables
- gross wage reduction amount: Simulates reductions in the average wage due to policy or economic crises.
- gross wage reduction year: Specifies the year when a reduction in average wages occurs.
- pension reduction amount: Models a reduction in average pension for specific years.
- reduction year: Identifies the year when a pension reduction is implemented.
'Variables for measurable metrics
- ratio of workers to pensioners